Free fall is a special case of uniformly accelerated linear motion. The object starts moving from rest only due to gravity, and air resistance and buoyancy are neglected. Its initial velocity is equal to 0 and its acceleration is g=9.8m/s², in the vertical downward direction. It is sometimes possible to make g=10m/s² for convenient calculation.
Formula of Free Fall
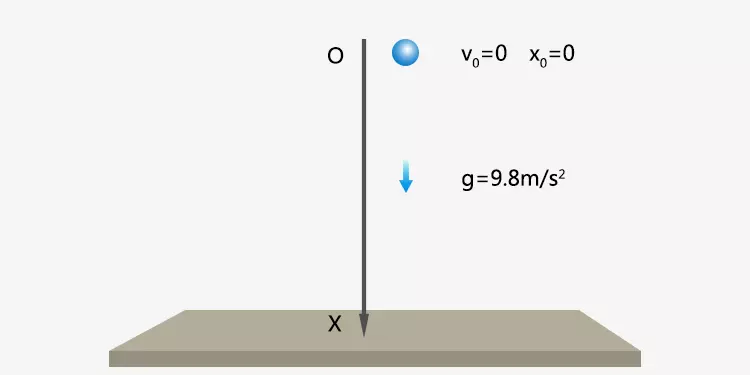
The x-axis is oriented vertically downward, and its origin is the starting point of the object. Then plug x₀ = 0, v₀ = 0 and a = g into the formula of uniformly accelerated linear motion.
Exercises on free fall are usually so simple that the answer can be derived by putting the numbers directly into the formula. Sometimes delayed release and non-particle objects are used to make the problem difficult.
Non-simultaneous free fall
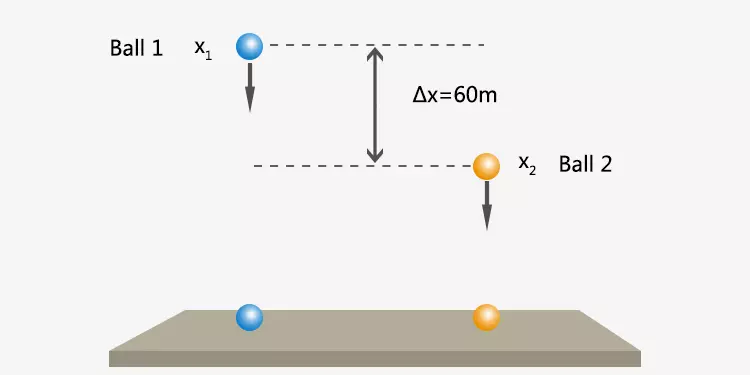
Two balls are falling freely due to gravity. Ball 1 is 60 m higher than ball 2. The ball 2 is released after 2 seconds the ball 1 is released and they reach the ground at the same time. What is their heights? Acceleration g is equal to 10 m/s² in order to ease the calculation.
The x₁ is the height of ball 1, the x₂ is the height of ball 2. The t₁ is the time taken by ball 1 to reach the ground. The t₂ is the time taken by ball 2 to reach the ground.
③-④
Non-point-like object falling
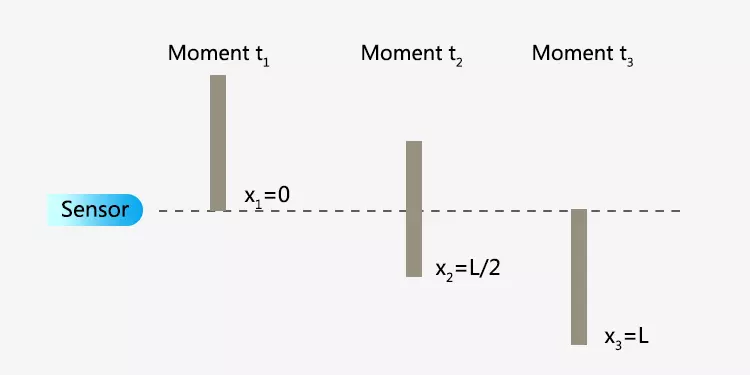
A wooden rod of length L falls freely due to gravity. Its head passes the sensor with a velocity v₁. When its tail passes the sensor, its velocity is v₃. What is the velocity of its middle part as it passes the sensor?
The length and shape of the object are used to interfere with your idea to make the problem more difficult. There is no rotation in this problem, only translation, and all the points of object are moving with the same velocity, so we only need to study one point on the object. We choose the top of rod to study its motion. Now let's translate this problem into another equivalent one. A freely falling particle passes through x₁ and x₃ with velocities v₁ and v₃ respectively, and find its velocity in the midpoint x₂.
We have already discussed this problem in the article about uniformly accelerated linear motion.


