If you are sitting in a moving ship and reading a book, the people on the ship think you are stationary. While the people on shore believe you are moving with the ship. Why would different people come to different conclusions about the same event. The reason is that they see the same problem from different perspectives: you are stationary with respect to the ship, but for the ground is in motion. Here the boat and ground are actually the reference frame. Before you can determine whether an object is moving, you need to choose an object as a reference frame, and then study its motion relative to the reference frame. The choice of reference frame is arbitrary, but you should choose those that will make your research easy. The ground is the most used reference frame in classical mechanics. Observing the same object in different reference frames will lead to different conclusions of motion.
In order to accurately describe the motion of an object, you also need to establish a coordinate system in the reference system. The creation of coordinate systems is arbitrary, but you should choose those that make the calculations easy. The most commonly used coordinate system is the Cartesian coordinate system. This system uses three mutually perpendicular axes to describe position: the X-axis, the Y-axis, and the Z-axis. These three axes intersect at a point called the origin whose coordinate is (0,0,0).

If an object is moving in one dimension, i.e., along a straight line, a straight number line is used to accurately express the object position. For example, a car moving on a straight section of highway.
If the object moves in two dimensions, i.e., in a plane, a rectangular coordinate system is needed to describe the object position. For example, a ship traveling in a lake.
When the object moves in three dimensions, a three-dimensional Cartesian coordinate system is needed to describe it. For example, an airplane flying in the air.
Once the coordinate system is established you can start to study the motion precisely. Position is the simplest and the first to be studied. It is defined as the location of an object with respect to the origin of coordinate system. It is usually represented by a set of coordinates (x, y, z), that is a vector: the object position can be described by coordinates along each axis.
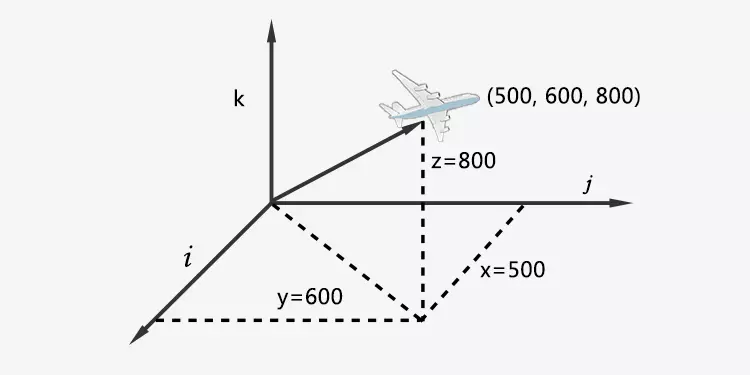
For example, if we take city A as the origin, the x-axis points east, the y-axis points north and the z-axis points vertically to the sky. An airplane is located 500km east of the city, 600km north of the city and at an altitude of 800m. Its coordinates are (500, 600, 800).