Difference between Instantaneous Speed & Instantaneous Velocity?

Instantaneous velocity

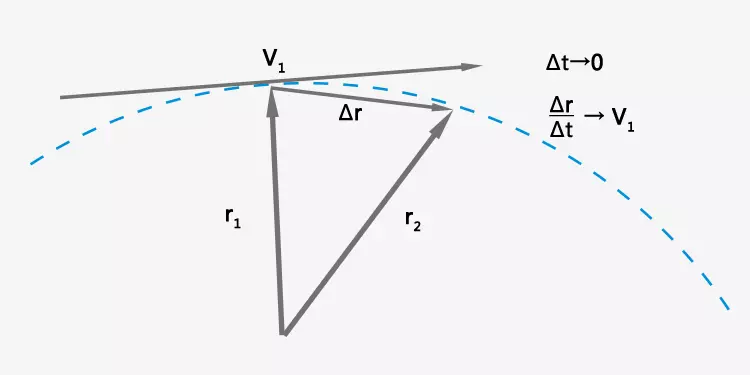
When Δt is finite, v represents the average velocity.

$v=\frac{\mathrm{\Delta r}}{\mathrm{\Delta t}}=\frac{\mathrm{r₂\; -\; r₁}}{\mathrm{t\; +\; \Delta t\; -\; t}}$

It is not accurate that we use the average velocity to represent the velocity at the moment t, because the velocity may vary in some cases. The average velocity cannot describe the motion of the object precisely, especially when we want to know the velocity at the moment t.

If we consider a shorter time interval or a smaller Δt, the smaller the difference between the average velocity and the velocity at moment t. You can imagine such a process: as Δt keeps decreasing, the difference between them becomes smaller and smaller; when Δt tends to zero, you can also interpret it as Δt is so very, very small so that the difference is negligible. Then the v calculated by the above formula is the instantaneous velocity at a certain moment. Instantaneous velocity is important in Newtonian physics because it helps determine the state of an object's motion at every moment.

$v=\frac{\mathrm{\Delta r}}{\mathrm{\Delta t}}\mathrm{(\Delta t\to 0)}$

Like the average velocity, the international unit of instantaneous velocity is the meter per second (m/s), while the other commonly used unit is the kilometer per hour (km/h). The direction of instantaneous velocity is the same as the direction of object motion at that moment.

Instantaneous speed

$v=\frac{s}{\mathrm{\Delta t}}$

Similar to the average velocity, a lot of information is lost when describing motion in terms of the average speed. For a more accurate description of motion, the average speed should be recorded for shorter intervals. If Δt tends to zero or is very, very small in the average speed formula, then v is the instantaneous speed at a given moment. It is a scalar that loses the information of direction. The international unit of instantaneous speed is the meter per second (m/s), and the other common unit is the kilometer per hour (km/h).

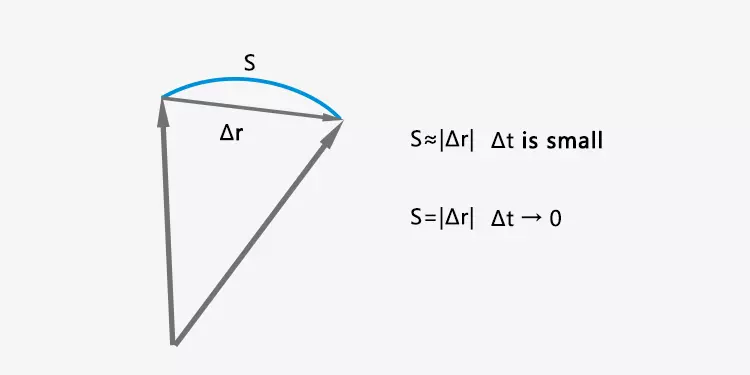
Due to the small time interval, the object can be considered to move along a straight line and without turning around. It means S=|Δr|, the distance is equal to the displacement magnitude. So the instantaneous speed is equal to the instantaneous velocity magnitude.